Mathematica

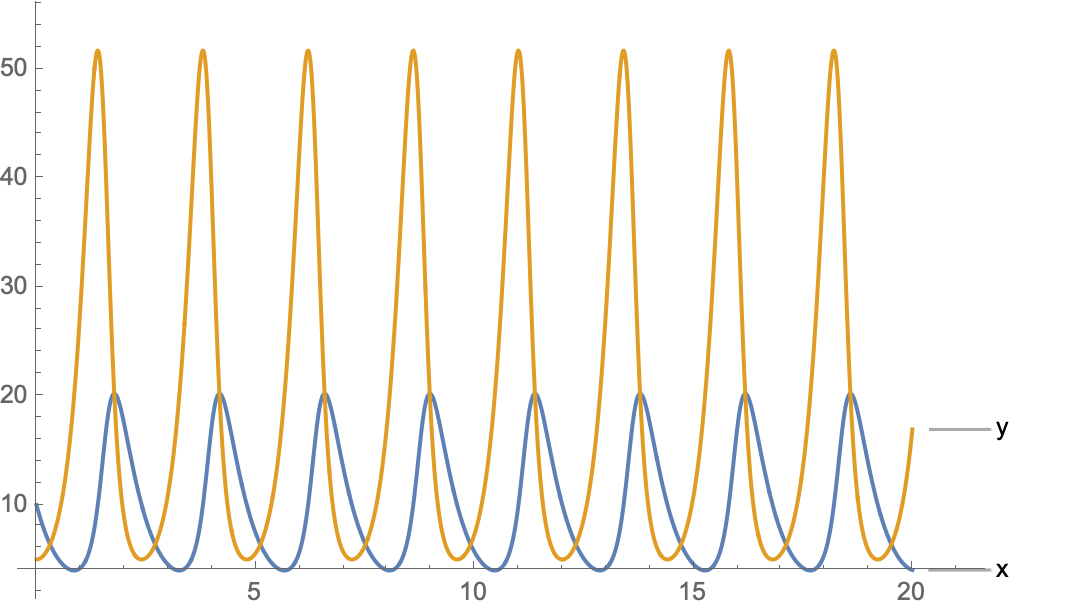
Lotka Volterra 方程 维基百科解释: Lotka Volterra 该方程组描述了 捕食系统模型 微分方程为: dx/dt = Axy - Bx dy/dt = Cy - Dxy => dy/dx = (Cy - Dxy) / (Axy - Bx) (1.1) {A, B, C, D} 均为模型系数 对微分方程(1.1)求解为: W 为Lambert W function 当系数为 {A -> 0.1, B -> 2, C -> 4, D -> 0.4, x -> {1,30}} y[x] 由于我们需要获得 x,y 关于自变量时间t，在模型系数{A,B,C,D}下的模型图，所以在Mathematica中对该方程组定义和设置模型系数 求该方程的数值解 创建Modelica模型 在0时刻 x[0] = 10, y[0] = 5 model = CreateSystemMod["Hare", Join[predatorPreyEq, {x[0] == 10, y[0] == 5}], t, Association[{"ParameterValues" -> params}]] 建模 Modelica 模型源码 ...

Why study Modelica I have seen a book “Model Thinking” before, and this time it happens to be in the CoVid-2019 disease ravaging the world, by chance I saw the prediction model SEIR about epidemiology, I took this opportunity to start studying mathematical models, and decided to pick up the mathematical analysis, ordinary differential equations, partial differential equations, differential geometry and other courses I studied in college. I came across the language Modelica, and since I had been studying Wolfram Mathematica, I was quite fond of SystemModeler of the Wolfram product line, so I started to buy the home edition of SystemModeler and purchased a book “Introduction and Improvement of Modelica Multi-Domain Physical System Modeling”. I began my exploration of Modelica with the expectation of gaining a deeper understanding of mathematical model building, using mathematical models to predict the future development pattern of an event and gaining more hidden information in quantitative data analysis. ...